• Two Chemicals A And B Are Combined To Form A Chemical C
• Two Chemicals A And B Are Combined To Form A Chemical C
• Two Chemicals A And B Are Combined To Form A Chemical C

$begingroup$

Chemicals A and B form an endothermic reaction, and chemicals C and D form an exothermic reaction. A Venn diagram is used to compare and contrast different topics. The items that are unique to each topic are represented in the separate sections of the circles, and the items that are common to both topics are represented in the center overlapping sections.

The problem says:

Two chemicals $A$ and $B$ are combined to form a chemical $C$. The rate, or velocity, of the reaction is proportional to the product of the instantaneous amounts of $A$ and $B$ not converted to chemical $C$. Initially, there are $40$ grams of $A$ and $50$ grams of $B$, and for each gram of $B$, $2$ grams of $A$ is used. It is observed that $10$ grams of $C$ is formed in $5$ minutes. How much is formed in $20$ minutes? What is the limiting amount of $C$ after a long time? How much of chemicals $A$ and $B$ remain after a long time?

$ A_0$ = 40 g, $ B_0$ = 50 g.

Well, first of all, $alpha $ = $ A_0 frac{M+N}{M} $ and $beta $ = $ B_0 frac{M+N}{N} $

and then our differential equation must be:

$frac{dX}{dt} = k(alpha-X)(beta-X) $ which can be easily solved. In order to create $ x$ part of the chemical C we will need 2 parts of $ A$ and one part of $ B$. This lead me to believe that $ M$ = 2 and $ N$ = 1By calculating $alpha$ = $ 40 frac{2+1}{2}$ = 60 and $beta $=$ 50 frac{2+1}{1}$ = 150. The differential equation must become $frac{dX}{dt} = k( 60-X)( 150-X) $ right?

I separate the variables and solved the equation$$int frac{dx}{(60-x)(150-x)}, = int kdt$$

$$ln frac{150-x}{60-x} = 90kt+C_1 $$By using X(0)=0, $$frac{150-x}{60-x} = Ce^{90k0}, C=frac{5}{2}$$and using X(5)=10 and solving for k, $$frac{150-10}{60-10} = frac{5}{2}e^{450k}, k= 2.5184X10^{-4}$$ and this is different to the solution of k in the solution manual which is $ k$ = $ 1.259X^{-4}$ and also, the differential equation is different, they obtain $frac{dX}{dt} = k( 120-2X)( 150-X)$

And I'm wondering why! I assume my mistake is in the values for $ M$ and $ N$. Can you give me a hand with this?

Rodrigo de Azevedo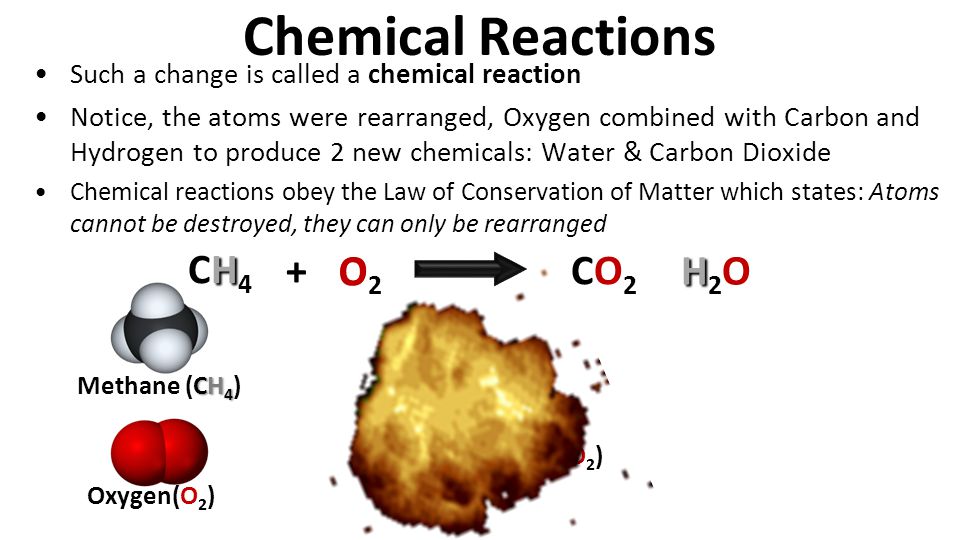$endgroup$

2 Answers

$begingroup$

The only difference is that your $k$ is their $2k$. And look, you get exactly double their answer. So you actually have the same answer.

Stephen Montgomery-SmithStephen Montgomery-Smith$endgroup$$begingroup$

Solving our equation for x: $$frac{150-x}{60-x}=C_1e^{90kt}$$ We obtain: $$X(t)=frac{60 C_1e^{90kt}-150}{C_1e^{90kt}-1}$$And if we substitute $ k=2.5184X10^{-4}$ and $ C_1 = frac{5}{2}$ this would be:$$ X(t)=frac{150 e^{0.0226656t}-150}{frac{5}{2}e^{0.0226656t}-1}$$Now we're able to answer Part A:$$ X(20)=frac{150 e^{0.0226656*20}-150}{frac{5}{2}e^{0.0226656*20}-1}=29.32$$ Part B:$$lim_{x to infty} f(x) = frac{150 e^{0.0226656t}-150}{frac{5}{2}e^{0.0226656t}-1} = 60g$$ <- This would be the maximum amount of C possible. And finally Part C:Chemical A remaining after a long time:$$A = A_0-frac{M}{M+N}(X) = 40-frac{2}{3}(60) = 0 g$$And Chemical B after a long time:$$B = B_0-frac{N}{M+N}(X) = 50-frac{1}{3}(60) = 30 g$$

Two Chemicals A And B Are Combined To Form A Chemical C

$endgroup$

Not the answer you're looking for? Browse other questions tagged ordinary-differential-equationschemistry or ask your own question.

Sets of elements can be combined or changed by using set operations. Much like addition or subtraction of real numbers, set operations are strictly defined to do something to the sets involved. The set operations are union, intersection, and complement:

Two Chemicals A And B Are Combined To Form A Chemical C

• The union of two sets A and B asks for all the elements in sets A and B — all of them together (without repeating any elements that they share).

• The intersection of the two sets A and B asks for all the elements that A and B have in common. If the two sets have nothing in common, then your answer is the empty set or null set.

• The complement of a set A asks for all the elements that aren’t in the set but are in the universal set. The universal set is everything under consideration at the time. For instance, if you’re working on sets that contain the letters of the English alphabet, then the universal set is all 26 letters.

Sample questions

1. Given the sets A = {2, 4, 6, 8}, B = {4, 8, 16, 24, 32}, C = {3, 6, 9, 12, 15, 18, 21}, and the universal set is

   The union of A and B consists of all the elements in A and B both, so

   Notice that the 4 and 8 aren’t repeated. The intersection of A and B consists of all the elements the two sets share, so

   The intersection of B and C is all the elements that the two sets share, but the two sets have nothing in common, so

   The complement of set C consists of everything that’s in the universal set that’s not in set C, so

2. Given the sets D = {0, 1, 2, 3, 4}, E = {0, 2, 6, 10}, F = {1, 3, 6, 10}, and U = {0, 1, 2, 3, … , 10}, find the sets

   
   

The parentheses in these set operation problems work the same way as parentheses in algebraic expressions — you perform what’s inside the parentheses first. To find the complement of the intersection of sets D and F, you first find the intersection D and F = {1, 3}; then, referring back to the universal set, U, you find the complement:

The complement is everything except the 1 and 3 in the intersection. To find the intersection of the two unions, first find the two unions: the union of D and E = {0, 1, 2, 3, 4, 6, 10}, and the union of E and F = {0, 1, 2, 3, 6, 10}. You write the intersection — what the two results have in common — as

Practice questions

1. 1.Given the sets A = {0, 2, 4, 6, 8, … , 20}, B = {0, 5, 10, 15, 20}, C = {7, 11, 17}, and the universal set U = {0, 1, 2, 3, 4, … , 20}, find the intersection ofA and B.

2. 2.Given the sets A = {0, 2, 4, 6, 8, … , 20}, B = {0, 5, 10, 15, 20}, C = {7, 11, 17}, and the universal set U = {0, 1, 2, 3, 4, … , 20}, find the union of A and B.

Two Chemicals A And B Are Combined To Form A Chemical C

Following are answers to the practice questions:

1. The answer is {0, 10, 20}.

   The sets A and B share only these three elements.

2. The answer is {0, 2, 4, 5, 6, 8, 10, 12, 14, 15, 16, 18, 20}.

   The union of A and B contains everything from A — all the even numbers from 0 to 20 — and everything from B — the multiples of 5 from 0 to 20. Essentially, you just list the even numbers and insert the 5 and 15 from set B. The 0 and 10 and 20 are already accounted for.